Difference between revisions of "File:Sutralomap.jpg"
(Importing image file) |
|||
| Line 1: | Line 1: | ||
| + | Overlappings of [[complex map]] of function |
||
| − | Importing image file |
||
| + | |||
| + | $z \mapsto -\ln(-z)$ |
||
| + | |||
| + | with two its approximations with entire functions, |
||
| + | |||
| + | $f_2(z)=\mathrm{SuTra}(2z) + \ln(2)$ , left, and |
||
| + | |||
| + | $f_4(z)=\mathrm{SuTra}(4z) + \ln(4)$ , right. |
||
| + | |||
| + | for function $f$, levels are shown with |
||
| + | |||
| + | $u\!+\!\mathrm i v= f(x\!+\!\mathrm i y)$ |
||
| + | |||
| + | in the $x$, $y$ plane. |
||
| + | |||
| + | This image is used as figure 20.7 of the Book |
||
| + | [[Superfunction]] (In Russian, 2014; the English version is in preparation, 2015) |
||
| + | <ref> |
||
| + | https://www.morebooks.de/store/ru/book/Суперфункции/isbn/978-3-659-56202-0 <br> |
||
| + | http://www.ils.uec.ac.jp/~dima/BOOK/202.pdf <br> |
||
| + | http://mizugadro.mydns.jp/BOOK/202.pdf |
||
| + | Д.Кузнецов. Суперфункции. Lambert Academic Publishing, 2014 |
||
| + | </ref>. |
||
| + | |||
| + | Function [[SuTra]] |
||
| + | as entire function with logarithmic asymptotic is described also in year 2013 at Hikari |
||
| + | <ref> |
||
| + | http://www.m-hikari.com/ams/ams-2013/ams-129-132-2013/kouznetsovAMS129-132-2013.pdf D.Kouznetsov. Entire Function with Logarithmic Asymptotic. Applied Mathematical Sciences, Vol. 7, 2013, no. 131, 6527 - 6541 |
||
| + | </ref>. |
||
| + | |||
| + | ==References== |
||
| + | <references/> |
||
| + | |||
| + | |||
| + | ==[[C++]] generator of map of $z\mapsto -\ln(-z)$ == |
||
| + | |||
| + | Files [[ado.cin]], [[conto.cin]] |
||
| + | should be loaded to the working directory in order to compile the code below |
||
| + | <poem><nomathjax><nowiki> |
||
| + | #include <math.h> |
||
| + | #include <stdio.h> |
||
| + | #include <stdlib.h> |
||
| + | #define DB double |
||
| + | #define DO(x,y) for(x=0;x<y;x++) |
||
| + | // using namespace std; |
||
| + | #include<complex> |
||
| + | typedef std::complex<double> z_type; |
||
| + | #define Re(x) x.real() |
||
| + | #define Im(x) x.imag() |
||
| + | #define I z_type(0.,1.) |
||
| + | #include "conto.cin" |
||
| + | //#include "tania.cin" |
||
| + | //#include "LambertW.cin" |
||
| + | //#include "SuZex.cin" |
||
| + | //#include "AuZex.cin" |
||
| + | //z_type tra(z_type z) {return z+exp(z);} |
||
| + | // z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z)); |
||
| + | //z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z)); |
||
| + | // return tra(sutra(z-1.));} |
||
| + | |||
| + | #include"sutran.cin" |
||
| + | |||
| + | int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd; |
||
| + | //DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar(); |
||
| + | int M=1001,M1=M+1; |
||
| + | int N=1001,N1=N+1; |
||
| + | DB X[M1],Y[N1]; |
||
| + | DB *g, *f, *w; // w is working array. |
||
| + | g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | char v[M1*N1]; // v is working array |
||
| + | //FILE *o;o=fopen("sutranmap.eps","w"); ado(o,2002,2002); |
||
| + | FILE *o;o=fopen("mlogmap.eps","w"); ado(o,2002,2002); |
||
| + | fprintf(o,"1001 1001 translate\n 100 100 scale\n"); |
||
| + | fprintf(o,"1 setlinejoin 2 setlinecap\n"); |
||
| + | DO(m,M1) X[m]=-10+.02*(m-.5); |
||
| + | DO(n,N1) Y[n]=-10+.02*(n-.5); |
||
| + | //for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); } |
||
| + | for(m=-10;m<11;m++){M(m,-10) L(m,10) } |
||
| + | for(n=-10;n<11;n++){M( -10,n) L(10,n)} |
||
| + | fprintf(o,".006 W 0 0 0 RGB S\n"); |
||
| + | DO(m,M1)DO(n,N1){ g[m*N1+n]=999; |
||
| + | f[m*N1+n]=999;} |
||
| + | DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x); |
||
| + | DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019) |
||
| + | // c=AuZex01(z-1.); |
||
| + | // c=AuZexAsy(LambertW(z))+1.; |
||
| + | //c=log(suzex(z)); |
||
| + | // c=sutran(z); |
||
| + | c=-log(-z); |
||
| + | // p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p; |
||
| + | p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;} |
||
| + | }} |
||
| + | fprintf(o,"1 setlinejoin 1 setlinecap\n"); |
||
| + | p=1.2;q=.4; |
||
| + | /* p=9;q=.16; |
||
| + | conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n"); |
||
| + | */ |
||
| + | for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n"); |
||
| + | conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n"); |
||
| + | for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n"); |
||
| + | // fprintf(o,"0 setlinejoin 0 setlinecap\n"); |
||
| + | // M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n"); |
||
| + | //#include "plofu.cin" |
||
| + | fprintf(o,"showpage\n"); |
||
| + | fprintf(o,"%c%cTrailer\n",'%','%'); |
||
| + | fclose(o); free(f); free(g); free(w); |
||
| + | system("epstopdf mlogmap.eps"); |
||
| + | system( "open mlogmap.pdf"); //for macintosh |
||
| + | getchar(); system("killall Preview"); // For macintosh |
||
| + | } |
||
| + | </nowiki></nomathjax></poem> |
||
| + | |||
| + | ==[[C++]] generator of map of $f_2$ == |
||
| + | |||
| + | Files [[ado.cin]], |
||
| + | [[conto.cin]], |
||
| + | [[sutran.cin]] |
||
| + | should be loaded to the working directory in order to compile the code below |
||
| + | <poem><nomathjax><nowiki> |
||
| + | #include <math.h> |
||
| + | #include <stdio.h> |
||
| + | #include <stdlib.h> |
||
| + | #define DB double |
||
| + | #define DO(x,y) for(x=0;x<y;x++) |
||
| + | //using namespace std; |
||
| + | #include<complex> |
||
| + | typedef std::complex<double> z_type; |
||
| + | #define Re(x) x.real() |
||
| + | #define Im(x) x.imag() |
||
| + | #define I z_type(0.,1.) |
||
| + | #include "conto.cin" |
||
| + | //#include "tania.cin" |
||
| + | //#include "LambertW.cin" |
||
| + | //#include "SuZex.cin" |
||
| + | //#include "AuZex.cin" |
||
| + | //z_type tra(z_type z) {return z+exp(z);} |
||
| + | // z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z)); |
||
| + | //z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z)); |
||
| + | // return tra(sutra(z-1.));} |
||
| + | |||
| + | #include"sutran.cin" |
||
| + | |||
| + | int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd; |
||
| + | //DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar(); |
||
| + | int M=1001,M1=M+1; |
||
| + | int N=1001,N1=N+1; |
||
| + | DB X[M1],Y[N1]; |
||
| + | DB *g, *f, *w; // w is working array. |
||
| + | g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | char v[M1*N1]; // v is working array |
||
| + | FILE *o;o=fopen("sutra2map.eps","w"); ado(o,2002,2002); |
||
| + | fprintf(o,"1001 1001 translate\n 100 100 scale\n"); |
||
| + | fprintf(o,"1 setlinejoin 2 setlinecap\n"); |
||
| + | DO(m,M1) X[m]=-10+.02*(m-.5); |
||
| + | DO(n,N1) Y[n]=-10+.02*(n-.5); |
||
| + | //for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); } |
||
| + | for(m=-10;m<11;m++){M(m,-10) L(m,10) } |
||
| + | for(n=-10;n<11;n++){M( -10,n) L(10,n)} |
||
| + | fprintf(o,".006 W 0 0 0 RGB S\n"); |
||
| + | DO(m,M1)DO(n,N1){ g[m*N1+n]=999; |
||
| + | f[m*N1+n]=999;} |
||
| + | DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x); |
||
| + | DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019) |
||
| + | // c=AuZex01(z-1.); |
||
| + | // c=AuZexAsy(LambertW(z))+1.; |
||
| + | //c=log(suzex(z)); |
||
| + | c=sutran(2.*z)+log(2.); |
||
| + | // p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p; |
||
| + | p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;} |
||
| + | }} |
||
| + | fprintf(o,"1 setlinejoin 1 setlinecap\n"); |
||
| + | p=1.2;q=.4; |
||
| + | /* p=9;q=.16; |
||
| + | conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n"); |
||
| + | */ |
||
| + | for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n"); |
||
| + | conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n"); |
||
| + | for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n"); |
||
| + | // fprintf(o,"0 setlinejoin 0 setlinecap\n"); |
||
| + | // M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n"); |
||
| + | //#include "plofu.cin" |
||
| + | fprintf(o,"showpage\n"); |
||
| + | fprintf(o,"%c%cTrailer\n",'%','%'); |
||
| + | fclose(o); free(f); free(g); free(w); |
||
| + | system("epstopdf sutra2map.eps"); |
||
| + | system( "open sutra2map.pdf"); //for macintosh |
||
| + | getchar(); system("killall Preview"); // For macintosh |
||
| + | } |
||
| + | </nowiki></nomathjax></poem> |
||
| + | |||
| + | ==[[C++]] generator of map of $f_4$== |
||
| + | |||
| + | Files [[ado.cin]], |
||
| + | [[conto.cin]], |
||
| + | [[sutran.cin]] |
||
| + | should be loaded to the working directory in order to compile the code below |
||
| + | <poem><nomathjax><nowiki> |
||
| + | #include <math.h> |
||
| + | #include <stdio.h> |
||
| + | #include <stdlib.h> |
||
| + | #define DB double |
||
| + | #define DO(x,y) for(x=0;x<y;x++) |
||
| + | //using namespace std; |
||
| + | #include<complex> |
||
| + | typedef std::complex<double> z_type; |
||
| + | #define Re(x) x.real() |
||
| + | #define Im(x) x.imag() |
||
| + | #define I z_type(0.,1.) |
||
| + | #include "conto.cin" |
||
| + | //#include "tania.cin" |
||
| + | //#include "LambertW.cin" |
||
| + | //#include "SuZex.cin" |
||
| + | //#include "AuZex.cin" |
||
| + | //z_type tra(z_type z) {return z+exp(z);} |
||
| + | // z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z)); |
||
| + | //z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z)); |
||
| + | // return tra(sutra(z-1.));} |
||
| + | |||
| + | #include"sutran.cin" |
||
| + | |||
| + | int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd; |
||
| + | //DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar(); |
||
| + | int M=1001,M1=M+1; |
||
| + | int N=1001,N1=N+1; |
||
| + | DB X[M1],Y[N1]; |
||
| + | DB *g, *f, *w; // w is working array. |
||
| + | g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB))); |
||
| + | char v[M1*N1]; // v is working array |
||
| + | FILE *o;o=fopen("sutra4map.eps","w"); ado(o,2002,2002); |
||
| + | fprintf(o,"1001 1001 translate\n 100 100 scale\n"); |
||
| + | fprintf(o,"1 setlinejoin 2 setlinecap\n"); |
||
| + | DO(m,M1) X[m]=-10+.02*(m-.5); |
||
| + | DO(n,N1) Y[n]=-10+.02*(n-.5); |
||
| + | //for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); } |
||
| + | for(m=-10;m<11;m++){M(m,-10) L(m,10) } |
||
| + | for(n=-10;n<11;n++){M( -10,n) L(10,n)} |
||
| + | fprintf(o,".006 W 0 0 0 RGB S\n"); |
||
| + | DO(m,M1)DO(n,N1){ g[m*N1+n]=999; |
||
| + | f[m*N1+n]=999;} |
||
| + | DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x); |
||
| + | DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019) |
||
| + | // c=AuZex01(z-1.); |
||
| + | // c=AuZexAsy(LambertW(z))+1.; |
||
| + | //c=log(suzex(z)); |
||
| + | c=sutran(4.*z)+log(4.); |
||
| + | // p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p; |
||
| + | p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;} |
||
| + | }} |
||
| + | fprintf(o,"1 setlinejoin 1 setlinecap\n"); |
||
| + | p=1.2;q=.4; |
||
| + | /* p=9;q=.16; |
||
| + | conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n"); |
||
| + | conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n"); |
||
| + | */ |
||
| + | for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n"); |
||
| + | for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n"); |
||
| + | for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n"); |
||
| + | conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n"); |
||
| + | for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n"); |
||
| + | // fprintf(o,"0 setlinejoin 0 setlinecap\n"); |
||
| + | // M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n"); |
||
| + | //#include "plofu.cin" |
||
| + | fprintf(o,"showpage\n"); |
||
| + | fprintf(o,"%c%cTrailer\n",'%','%'); |
||
| + | fclose(o); free(f); free(g); free(w); |
||
| + | system("epstopdf sutra4map.eps"); |
||
| + | system( "open sutra4map.pdf"); //for macintosh |
||
| + | getchar(); system("killall Preview"); // For macintosh |
||
| + | } |
||
| + | </nowiki></nomathjax></poem> |
||
| + | |||
| + | ==[[Latex]] combiner== |
||
| + | |||
| + | <poem><nomathjax><nowiki> |
||
| + | \documentclass[12pt]{article} |
||
| + | \paperwidth 1216px |
||
| + | \paperheight 602px |
||
| + | \textwidth 1380px |
||
| + | \textheight 1300px |
||
| + | \topmargin -106px |
||
| + | \oddsidemargin -71px |
||
| + | \usepackage{graphics} |
||
| + | \usepackage{rotating} |
||
| + | \newcommand \sx {\scalebox} |
||
| + | \newcommand \rot {\begin{rotate}} |
||
| + | \newcommand \ero {\end{rotate}} |
||
| + | \newcommand \ing {\includegraphics} |
||
| + | \newcommand \rmi {\mathrm{i}} |
||
| + | \begin{document} |
||
| + | \parindent 0pt |
||
| + | \sx{.3}{\begin{picture}(2000,2002) |
||
| + | \put(30,1950){\sx{8}{$y$}} |
||
| + | \put(30,1780){\sx{7}{$8$}} |
||
| + | \put(30,1580){\sx{7}{$6$}} |
||
| + | \put(30,1380){\sx{7}{$4$}} |
||
| + | \put(30,1180){\sx{7}{$2$}} |
||
| + | \put(30,0980){\sx{7}{$0$}} |
||
| + | \put(-22,780){\sx{7}{$-2$}} |
||
| + | \put(-22,580){\sx{7}{$-4$}} |
||
| + | \put(-22,380){\sx{7}{$-6$}} |
||
| + | \put(-22,180){\sx{7}{$-8$}} |
||
| + | \put(120, 6){\sx{7}{$-8$}} |
||
| + | \put(320, 6){\sx{7}{$-6$}} |
||
| + | \put(520, 6){\sx{7}{$-4$}} |
||
| + | \put(720, 6){\sx{7}{$-2$}} |
||
| + | \put(988, 6){\sx{7}{$0$}} |
||
| + | \put(1188, 6){\sx{7}{$2$}} |
||
| + | \put(1388, 6){\sx{7}{$4$}} |
||
| + | \put(1588, 6){\sx{7}{$6$}} |
||
| + | \put(1788, 6){\sx{7}{$8$}} |
||
| + | \put(1958, 6){\sx{8}{$x$}} |
||
| + | %\put(0,0){\ing{SuTraMap}} |
||
| + | \put(0,0){\ing{sutra2map}} |
||
| + | \put(0,0){\ing{mlogmap}} |
||
| + | %\zoomax % |
||
| + | \put(200,1414){\sx{7}{\rot{56}$u\!=\!-2.2$\ero}} |
||
| + | \put(352,1328){\sx{7}{\rot{56}$u\!=\!-2$\ero}} |
||
| + | \put(462,1238){\sx{7}{\rot{56}$u\!=\!-1.8$\ero}} |
||
| + | \put(1277,1590){\sx{7}{\rot{68}$v\!=\!2$\ero}} |
||
| + | \put(596,1632){\sx{7}{\rot{-55}$v\!=\!1$\ero}} |
||
| + | \put(340,1128){\sx{7}{\rot{-10}$v\!=\!0.2$\ero}} |
||
| + | \put(310,0984){\sx{7}{$v\!=\!0$}} |
||
| + | \put(330,0832){\sx{7}{\rot{10}$v\!=\!-0.2$\ero}} |
||
| + | \put(620,0332){\sx{7}{\rot{55}$v\!=\!-1$\ero}} |
||
| + | \put(1209,488){\sx{7}{\rot{-70}$v\!=\!-2$\ero}} |
||
| + | %\put(1490,772){\sx{7}{\rot{-11}$v\!=\!-3$\ero}} |
||
| + | \end{picture}} |
||
| + | \rule{8pt}{0pt} |
||
| + | %\sx{.12}{\begin{picture}(2002,2002) |
||
| + | \sx{.3}{\begin{picture}(2000,2002) |
||
| + | %\put(40,20){\ing{b271tMap3}} |
||
| + | %\put(40,20){\ing{ExpMap}} |
||
| + | \put(30,1950){\sx{8}{$y$}} |
||
| + | \put(30,1780){\sx{7}{$8$}} |
||
| + | \put(30,1580){\sx{7}{$6$}} |
||
| + | \put(30,1380){\sx{7}{$4$}} |
||
| + | \put(30,1180){\sx{7}{$2$}} |
||
| + | \put(30,0980){\sx{7}{$0$}} |
||
| + | \put(-22,780){\sx{7}{$-2$}} |
||
| + | \put(-22,580){\sx{7}{$-4$}} |
||
| + | \put(-22,380){\sx{7}{$-6$}} |
||
| + | \put(-22,180){\sx{7}{$-8$}} |
||
| + | \put(120, 6){\sx{7}{$-8$}} |
||
| + | \put(320, 6){\sx{7}{$-6$}} |
||
| + | \put(520, 6){\sx{7}{$-4$}} |
||
| + | \put(720, 6){\sx{7}{$-2$}} |
||
| + | \put(988, 6){\sx{7}{$0$}} |
||
| + | \put(1188, 6){\sx{7}{$2$}} |
||
| + | \put(1388, 6){\sx{7}{$4$}} |
||
| + | \put(1588, 6){\sx{7}{$6$}} |
||
| + | \put(1788, 6){\sx{7}{$8$}} |
||
| + | \put(1958, 6){\sx{8}{$x$}} |
||
| + | %\put(0,0){\ing{SuTraMap}} |
||
| + | \put(0,0){\ing{sutra4map}} |
||
| + | \put(0,0){\ing{mlogmap}} |
||
| + | %\zoomax % |
||
| + | \put(200,1414){\sx{7}{\rot{56}$u\!=\!-2.2$\ero}} |
||
| + | \put(352,1328){\sx{7}{\rot{56}$u\!=\!-2$\ero}} |
||
| + | \put(462,1238){\sx{7}{\rot{56}$u\!=\!-1.8$\ero}} |
||
| + | \put(1277,1590){\sx{7}{\rot{68}$v\!=\!2$\ero}} |
||
| + | \put(596,1632){\sx{7}{\rot{-55}$v\!=\!1$\ero}} |
||
| + | \put(340,1128){\sx{7}{\rot{-10}$v\!=\!0.2$\ero}} |
||
| + | \put(310,0984){\sx{7}{$v\!=\!0$}} |
||
| + | \put(330,0832){\sx{7}{\rot{10}$v\!=\!-0.2$\ero}} |
||
| + | \put(620,0332){\sx{7}{\rot{55}$v\!=\!-1$\ero}} |
||
| + | \put(1209,488){\sx{7}{\rot{-70}$v\!=\!-2$\ero}} |
||
| + | %\put(1490,772){\sx{7}{\rot{-11}$v\!=\!-3$\ero}} |
||
| + | \end{picture}} |
||
| + | \end{document} |
||
| + | |||
| + | </nowiki></nomathjax></poem> |
||
| + | |||
| + | [[Category:Book]] |
||
| + | [[Category:BookMap]] |
||
| + | [[Category:Complex map]] |
||
| + | [[Category:C++]] |
||
| + | [[Category:Elementary function]] |
||
| + | [[Category:Latex]] |
||
| + | [[Category:Superfunction]] |
||
| + | [[Category:SuTra]] |
||
| + | [[Category:Trappmann function]] |
||
Latest revision as of 08:53, 1 December 2018
Overlappings of complex map of function
$z \mapsto -\ln(-z)$
with two its approximations with entire functions,
$f_2(z)=\mathrm{SuTra}(2z) + \ln(2)$ , left, and
$f_4(z)=\mathrm{SuTra}(4z) + \ln(4)$ , right.
for function $f$, levels are shown with
$u\!+\!\mathrm i v= f(x\!+\!\mathrm i y)$
in the $x$, $y$ plane.
This image is used as figure 20.7 of the Book Superfunction (In Russian, 2014; the English version is in preparation, 2015) [1].
Function SuTra as entire function with logarithmic asymptotic is described also in year 2013 at Hikari [2].
References
- ↑
https://www.morebooks.de/store/ru/book/Суперфункции/isbn/978-3-659-56202-0
http://www.ils.uec.ac.jp/~dima/BOOK/202.pdf
http://mizugadro.mydns.jp/BOOK/202.pdf Д.Кузнецов. Суперфункции. Lambert Academic Publishing, 2014 - ↑ http://www.m-hikari.com/ams/ams-2013/ams-129-132-2013/kouznetsovAMS129-132-2013.pdf D.Kouznetsov. Entire Function with Logarithmic Asymptotic. Applied Mathematical Sciences, Vol. 7, 2013, no. 131, 6527 - 6541
C++ generator of map of $z\mapsto -\ln(-z)$
Files ado.cin, conto.cin should be loaded to the working directory in order to compile the code below
#include <math.h>
#include <stdio.h>
#include <stdlib.h>
#define DB double
#define DO(x,y) for(x=0;x<y;x++)
// using namespace std;
#include<complex>
typedef std::complex<double> z_type;
#define Re(x) x.real()
#define Im(x) x.imag()
#define I z_type(0.,1.)
#include "conto.cin"
//#include "tania.cin"
//#include "LambertW.cin"
//#include "SuZex.cin"
//#include "AuZex.cin"
//z_type tra(z_type z) {return z+exp(z);}
// z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z));
//z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z));
// return tra(sutra(z-1.));}
#include"sutran.cin"
int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd;
//DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar();
int M=1001,M1=M+1;
int N=1001,N1=N+1;
DB X[M1],Y[N1];
DB *g, *f, *w; // w is working array.
g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
char v[M1*N1]; // v is working array
//FILE *o;o=fopen("sutranmap.eps","w"); ado(o,2002,2002);
FILE *o;o=fopen("mlogmap.eps","w"); ado(o,2002,2002);
fprintf(o,"1001 1001 translate\n 100 100 scale\n");
fprintf(o,"1 setlinejoin 2 setlinecap\n");
DO(m,M1) X[m]=-10+.02*(m-.5);
DO(n,N1) Y[n]=-10+.02*(n-.5);
//for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); }
for(m=-10;m<11;m++){M(m,-10) L(m,10) }
for(n=-10;n<11;n++){M( -10,n) L(10,n)}
fprintf(o,".006 W 0 0 0 RGB S\n");
DO(m,M1)DO(n,N1){ g[m*N1+n]=999;
f[m*N1+n]=999;}
DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x);
DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019)
// c=AuZex01(z-1.);
// c=AuZexAsy(LambertW(z))+1.;
//c=log(suzex(z));
// c=sutran(z);
c=-log(-z);
// p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p;
p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;}
}}
fprintf(o,"1 setlinejoin 1 setlinecap\n");
p=1.2;q=.4;
/* p=9;q=.16;
conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n");
*/
for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n");
conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n");
for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n");
// fprintf(o,"0 setlinejoin 0 setlinecap\n");
// M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n");
//#include "plofu.cin"
fprintf(o,"showpage\n");
fprintf(o,"%c%cTrailer\n",'%','%');
fclose(o); free(f); free(g); free(w);
system("epstopdf mlogmap.eps");
system( "open mlogmap.pdf"); //for macintosh
getchar(); system("killall Preview"); // For macintosh
}
C++ generator of map of $f_2$
Files ado.cin, conto.cin, sutran.cin should be loaded to the working directory in order to compile the code below
#include <math.h>
#include <stdio.h>
#include <stdlib.h>
#define DB double
#define DO(x,y) for(x=0;x<y;x++)
//using namespace std;
#include<complex>
typedef std::complex<double> z_type;
#define Re(x) x.real()
#define Im(x) x.imag()
#define I z_type(0.,1.)
#include "conto.cin"
//#include "tania.cin"
//#include "LambertW.cin"
//#include "SuZex.cin"
//#include "AuZex.cin"
//z_type tra(z_type z) {return z+exp(z);}
// z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z));
//z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z));
// return tra(sutra(z-1.));}
#include"sutran.cin"
int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd;
//DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar();
int M=1001,M1=M+1;
int N=1001,N1=N+1;
DB X[M1],Y[N1];
DB *g, *f, *w; // w is working array.
g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
char v[M1*N1]; // v is working array
FILE *o;o=fopen("sutra2map.eps","w"); ado(o,2002,2002);
fprintf(o,"1001 1001 translate\n 100 100 scale\n");
fprintf(o,"1 setlinejoin 2 setlinecap\n");
DO(m,M1) X[m]=-10+.02*(m-.5);
DO(n,N1) Y[n]=-10+.02*(n-.5);
//for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); }
for(m=-10;m<11;m++){M(m,-10) L(m,10) }
for(n=-10;n<11;n++){M( -10,n) L(10,n)}
fprintf(o,".006 W 0 0 0 RGB S\n");
DO(m,M1)DO(n,N1){ g[m*N1+n]=999;
f[m*N1+n]=999;}
DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x);
DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019)
// c=AuZex01(z-1.);
// c=AuZexAsy(LambertW(z))+1.;
//c=log(suzex(z));
c=sutran(2.*z)+log(2.);
// p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p;
p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;}
}}
fprintf(o,"1 setlinejoin 1 setlinecap\n");
p=1.2;q=.4;
/* p=9;q=.16;
conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n");
*/
for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n");
conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n");
for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n");
// fprintf(o,"0 setlinejoin 0 setlinecap\n");
// M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n");
//#include "plofu.cin"
fprintf(o,"showpage\n");
fprintf(o,"%c%cTrailer\n",'%','%');
fclose(o); free(f); free(g); free(w);
system("epstopdf sutra2map.eps");
system( "open sutra2map.pdf"); //for macintosh
getchar(); system("killall Preview"); // For macintosh
}
C++ generator of map of $f_4$
Files ado.cin, conto.cin, sutran.cin should be loaded to the working directory in order to compile the code below
#include <math.h>
#include <stdio.h>
#include <stdlib.h>
#define DB double
#define DO(x,y) for(x=0;x<y;x++)
//using namespace std;
#include<complex>
typedef std::complex<double> z_type;
#define Re(x) x.real()
#define Im(x) x.imag()
#define I z_type(0.,1.)
#include "conto.cin"
//#include "tania.cin"
//#include "LambertW.cin"
//#include "SuZex.cin"
//#include "AuZex.cin"
//z_type tra(z_type z) {return z+exp(z);}
// z_type sutra(z_type z){ if( Re(z)<2. || fabs(Im(z))>2. ) return log(suzex(z));
//z_type sutra(z_type z){ if( Re(z)<2. ) return log(suzex(z));
// return tra(sutra(z-1.));}
#include"sutran.cin"
int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd;
//DB x1=-1.1259817765745026; DO(n,8){ y=Re(suzex(x1)); x=y-1.; x1+=-1.2*x; printf("%18.16f %18.16f\n", x1,y);} getchar();
int M=1001,M1=M+1;
int N=1001,N1=N+1;
DB X[M1],Y[N1];
DB *g, *f, *w; // w is working array.
g=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
f=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
w=(DB *)malloc((size_t)((M1*N1)*sizeof(DB)));
char v[M1*N1]; // v is working array
FILE *o;o=fopen("sutra4map.eps","w"); ado(o,2002,2002);
fprintf(o,"1001 1001 translate\n 100 100 scale\n");
fprintf(o,"1 setlinejoin 2 setlinecap\n");
DO(m,M1) X[m]=-10+.02*(m-.5);
DO(n,N1) Y[n]=-10+.02*(n-.5);
//for(n=0;n<N1;n++) { Y[n]=1.09*sinh((3./200.)*(n-200)); printf("%3d %9.6f\n",n,Y[n]); }
for(m=-10;m<11;m++){M(m,-10) L(m,10) }
for(n=-10;n<11;n++){M( -10,n) L(10,n)}
fprintf(o,".006 W 0 0 0 RGB S\n");
DO(m,M1)DO(n,N1){ g[m*N1+n]=999;
f[m*N1+n]=999;}
DO(m,M1){x=X[m]; if(m/10*10==m) printf("x=%6.3f\n",x);
DO(n,N1){y=Y[n]; z=z_type(x,y); //if(abs(z+2.)>.019)
// c=AuZex01(z-1.);
// c=AuZexAsy(LambertW(z))+1.;
//c=log(suzex(z));
c=sutran(4.*z)+log(4.);
// p=abs(c-z)/(abs(c)+abs(z)); p=-log(p)/log(10.); if(p>0 && p<17) g[m*N1+n]=p;
p=Re(c); q=Im(c); if(p>-19 && p<19 && ( x<2. || (fabs(q)>1.e-12 && fabs(p)>1.e-12)) ){ g[m*N1+n]=p;f[m*N1+n]=q;}
}}
fprintf(o,"1 setlinejoin 1 setlinecap\n");
p=1.2;q=.4;
/* p=9;q=.16;
conto(o,g,w,v,X,Y,M,N,(15.3 ),-p,p); fprintf(o,".01 W .4 1 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(15. ),-p,p); fprintf(o,".02 W 1 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14.7 ),-p,p); fprintf(o,".01 W 1 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(14. ),-p,p); fprintf(o,".01 W .2 .2 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(13. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(12. ),-p,p); fprintf(o,".03 W 0 0 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(11. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N,(10. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (9. ),-p,p); fprintf(o,".03 W 0 1 1 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (8. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (7. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (6. ),-p,p); fprintf(o,".04 W 0 .5 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (5. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (4. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (3. ),-p,p); fprintf(o,".02 W 1 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (2. ),-p,p); fprintf(o,".01 W 0 0 0 RGB S\n");
conto(o,g,w,v,X,Y,M,N, (1. ),-p,p); fprintf(o,".02 W .5 0 0 RGB S\n");
*/
for(m=-8;m<8;m++)for(n=2;n<10;n+=2)conto(o,f,w,v,X,Y,M,N,(m+.1*n),-q,q);fprintf(o,".007 W 0 .6 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);fprintf(o,".007 W .9 0 0 RGB S\n");
for(m=0;m<8;m++) for(n=2;n<10;n+=2)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);fprintf(o,".007 W 0 0 .9 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".02 W .8 0 0 RGB S\n");
for(m= 1;m<17;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".02 W 0 0 .8 RGB S\n");
conto(o,f,w,v,X,Y,M,N, (0. ),-2*p,2*p); fprintf(o,".02 W .5 0 .5 RGB S\n");
for(m=-16;m<17;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".02 W 0 0 0 RGB S\n");
// fprintf(o,"0 setlinejoin 0 setlinecap\n");
// M(-10,0)L(0,0) fprintf(o,"1 1 1 RGB .02 W S\n");
//#include "plofu.cin"
fprintf(o,"showpage\n");
fprintf(o,"%c%cTrailer\n",'%','%');
fclose(o); free(f); free(g); free(w);
system("epstopdf sutra4map.eps");
system( "open sutra4map.pdf"); //for macintosh
getchar(); system("killall Preview"); // For macintosh
}
Latex combiner
\documentclass[12pt]{article}
\paperwidth 1216px
\paperheight 602px
\textwidth 1380px
\textheight 1300px
\topmargin -106px
\oddsidemargin -71px
\usepackage{graphics}
\usepackage{rotating}
\newcommand \sx {\scalebox}
\newcommand \rot {\begin{rotate}}
\newcommand \ero {\end{rotate}}
\newcommand \ing {\includegraphics}
\newcommand \rmi {\mathrm{i}}
\begin{document}
\parindent 0pt
\sx{.3}{\begin{picture}(2000,2002)
\put(30,1950){\sx{8}{$y$}}
\put(30,1780){\sx{7}{$8$}}
\put(30,1580){\sx{7}{$6$}}
\put(30,1380){\sx{7}{$4$}}
\put(30,1180){\sx{7}{$2$}}
\put(30,0980){\sx{7}{$0$}}
\put(-22,780){\sx{7}{$-2$}}
\put(-22,580){\sx{7}{$-4$}}
\put(-22,380){\sx{7}{$-6$}}
\put(-22,180){\sx{7}{$-8$}}
\put(120, 6){\sx{7}{$-8$}}
\put(320, 6){\sx{7}{$-6$}}
\put(520, 6){\sx{7}{$-4$}}
\put(720, 6){\sx{7}{$-2$}}
\put(988, 6){\sx{7}{$0$}}
\put(1188, 6){\sx{7}{$2$}}
\put(1388, 6){\sx{7}{$4$}}
\put(1588, 6){\sx{7}{$6$}}
\put(1788, 6){\sx{7}{$8$}}
\put(1958, 6){\sx{8}{$x$}}
%\put(0,0){\ing{SuTraMap}}
\put(0,0){\ing{sutra2map}}
\put(0,0){\ing{mlogmap}}
%\zoomax %
\put(200,1414){\sx{7}{\rot{56}$u\!=\!-2.2$\ero}}
\put(352,1328){\sx{7}{\rot{56}$u\!=\!-2$\ero}}
\put(462,1238){\sx{7}{\rot{56}$u\!=\!-1.8$\ero}}
\put(1277,1590){\sx{7}{\rot{68}$v\!=\!2$\ero}}
\put(596,1632){\sx{7}{\rot{-55}$v\!=\!1$\ero}}
\put(340,1128){\sx{7}{\rot{-10}$v\!=\!0.2$\ero}}
\put(310,0984){\sx{7}{$v\!=\!0$}}
\put(330,0832){\sx{7}{\rot{10}$v\!=\!-0.2$\ero}}
\put(620,0332){\sx{7}{\rot{55}$v\!=\!-1$\ero}}
\put(1209,488){\sx{7}{\rot{-70}$v\!=\!-2$\ero}}
%\put(1490,772){\sx{7}{\rot{-11}$v\!=\!-3$\ero}}
\end{picture}}
\rule{8pt}{0pt}
%\sx{.12}{\begin{picture}(2002,2002)
\sx{.3}{\begin{picture}(2000,2002)
%\put(40,20){\ing{b271tMap3}}
%\put(40,20){\ing{ExpMap}}
\put(30,1950){\sx{8}{$y$}}
\put(30,1780){\sx{7}{$8$}}
\put(30,1580){\sx{7}{$6$}}
\put(30,1380){\sx{7}{$4$}}
\put(30,1180){\sx{7}{$2$}}
\put(30,0980){\sx{7}{$0$}}
\put(-22,780){\sx{7}{$-2$}}
\put(-22,580){\sx{7}{$-4$}}
\put(-22,380){\sx{7}{$-6$}}
\put(-22,180){\sx{7}{$-8$}}
\put(120, 6){\sx{7}{$-8$}}
\put(320, 6){\sx{7}{$-6$}}
\put(520, 6){\sx{7}{$-4$}}
\put(720, 6){\sx{7}{$-2$}}
\put(988, 6){\sx{7}{$0$}}
\put(1188, 6){\sx{7}{$2$}}
\put(1388, 6){\sx{7}{$4$}}
\put(1588, 6){\sx{7}{$6$}}
\put(1788, 6){\sx{7}{$8$}}
\put(1958, 6){\sx{8}{$x$}}
%\put(0,0){\ing{SuTraMap}}
\put(0,0){\ing{sutra4map}}
\put(0,0){\ing{mlogmap}}
%\zoomax %
\put(200,1414){\sx{7}{\rot{56}$u\!=\!-2.2$\ero}}
\put(352,1328){\sx{7}{\rot{56}$u\!=\!-2$\ero}}
\put(462,1238){\sx{7}{\rot{56}$u\!=\!-1.8$\ero}}
\put(1277,1590){\sx{7}{\rot{68}$v\!=\!2$\ero}}
\put(596,1632){\sx{7}{\rot{-55}$v\!=\!1$\ero}}
\put(340,1128){\sx{7}{\rot{-10}$v\!=\!0.2$\ero}}
\put(310,0984){\sx{7}{$v\!=\!0$}}
\put(330,0832){\sx{7}{\rot{10}$v\!=\!-0.2$\ero}}
\put(620,0332){\sx{7}{\rot{55}$v\!=\!-1$\ero}}
\put(1209,488){\sx{7}{\rot{-70}$v\!=\!-2$\ero}}
%\put(1490,772){\sx{7}{\rot{-11}$v\!=\!-3$\ero}}
\end{picture}}
\end{document}
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 06:14, 1 December 2018 | 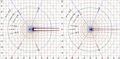 | 2,533 × 1,254 (1.26 MB) | Maintenance script (talk | contribs) | Importing image file |
You cannot overwrite this file.
File usage
There are no pages that use this file.