File:Tetma.jpg
Original file (3,341 × 1,675 pixels, file size: 1.89 MB, MIME type: image/jpeg)
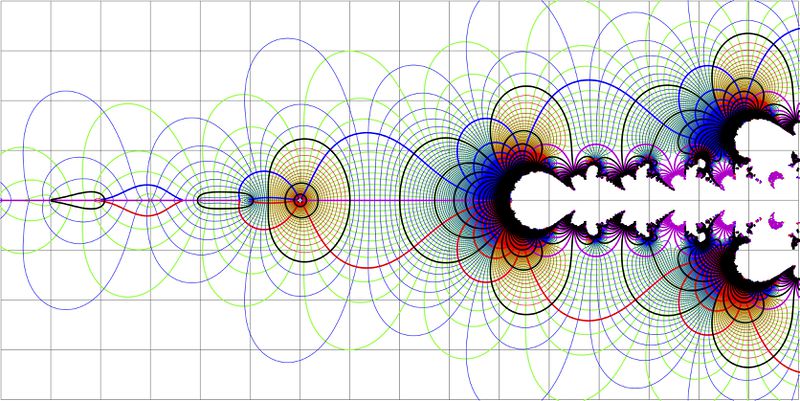
Complex map of the natural tetration without labels.
This image is loaded to the ingrampublishing.com
In order to use it in the book Суперфункции,
https://www.morebooks.de/store/ru/book/Суперфункции/isbn/978-3-659-56202-0
\url{http://mizugadro.mydns.jp/BOOK/201.pdf}
The low resolution draft also had been loaded at the site of ingrampublishing
C++ generator
Files ado.cin conto.cin fsexp.cin fslog.cin should be loaded in order to compile the code below
#include <math.h>
#include <stdio.h>
#include <stdlib.h>
#define DB double
#define DO(x,y) for(x=0;x<y;x++)
//using namespace std;
#include<complex>
typedef std::complex<double> z_type;
#define Re(x) x.real()
#define Im(x) x.imag()
#define I z_type(0.,1.)
#include "conto.cin"
#include "fsexp.cin"
//#include "fslog.cin"
int main(){ int j,k,m,n; DB x,y, p,q, t; z_type z,c,d, cu,cd;
int M=801,M1=M+1;
int N=401,N1=N+1;
DB X[M1],Y[N1], g[M1*N1],f[M1*N1], w[M1*N1]; // w is working array.
char v[M1*N1]; // v is working array
//FILE *o;o=fopen("b271t0.eps","w"); ado(o,87,87);
FILE *o;o=fopen("tetma.eps","w"); ado(o,1604,804);
fprintf(o,"802 402 translate\n 100 100 scale\n");
DO(m,M1) X[m]=-8.+.02*(m-.5);
DO(n,N1) Y[n]=-4.+.02*(n-.5);
for(m=-8;m<17;m++) {M(m,-4)L(m,4)}
for(n=-4;n<5;n++) {M( -8,n)L(8,n)} fprintf(o,"2 setlinecap .006 W 0 0 0 RGB S\n");
//fprintf(o,"/adobe-Roman findfont .6 scalefont setfont\n");
DO(m,M1)DO(n,N1){ g[m*N1+n]=9999;
f[m*N1+n]=9999;}
//DB b=sqrt(2);
DO(m,M1){x=X[m]; printf("x=%6.3f\n",x);
DO(n,N1){y=Y[n]; z=z_type(x,y); if(abs(z+2.)>.04)
{ c=FSEXP(z);
// c=FSLOG(z);
p=Re(c); q=Im(c);
if(p>-9999 && p<9999 && fabs(q)>1.e-12) g[m*N1+n]=p;
if(q>-9999 && q<9999 && fabs(q)>1.e-12) f[m*N1+n]=q;
}
}}
fprintf(o,"1 setlinejoin 2 setlinecap\n");
p=2.; q=1.1;;
//#include "plofu.cin"
p=2;q=1;
for(m=-19;m<19;m++)for(n=1;n<10;n+=1)conto(o,f,w,v,X,Y,M,N, (m+.1*n),-q,q);
fprintf(o,".02 W 0 1 0 RGB S\n");
for(m=0;m<29;m++) for(n=1;n<10;n+=1)conto(o,g,w,v,X,Y,M,N,-(m+.1*n),-q,q);
fprintf(o,".01 W 1 0 0 RGB S\n");
for(m=0;m<29;m++) for(n=1;n<10;n+=1)conto(o,g,w,v,X,Y,M,N, (m+.1*n),-q,q);
fprintf(o,".01 W 0 0 1 RGB S\n");
for(m= 1;m<20;m++) conto(o,f,w,v,X,Y,M,N, (0.-m),-p,p);fprintf(o,".03 W 1 0 0 RGB S\n");
for(m= 1;m<20;m++) conto(o,f,w,v,X,Y,M,N, (0.+m),-p,p);fprintf(o,".03 W 0 0 1 RGB S\n");
conto(o,f,w,v,X,Y,M,N, (0. ),-p,p); fprintf(o,".03 W .8 0 .8 RGB S\n");
for(m=-31;m<32;m++)conto(o,g,w,v,X,Y,M,N,(0.+m),-p,p);fprintf(o,".03 W 0 0 0 RGB S\n");
fprintf(o,"0 setlinejoin 0 setlinecap\n");
fprintf(o,"showpage\n");
fprintf(o,"%cTrailer\n",'%');
fclose(o);
system("epstopdf tetma.eps");
system( "open tetma.pdf"); //for macintosh
getchar(); system("killall Preview"); // For macintosh
References
http://www.ams.org/mcom/2009-78-267/S0025-5718-09-02188-7/home.html D.Kouznetsov. (2009). Solutions of F(z+1)=exp(F(z)) in the complex plane.. Mathematics of Computation, 78: 1647-1670. DOI:10.1090/S0025-5718-09-02188-7.
http://www.ils.uec.ac.jp/~dima/PAPERS/2010vladie.pdf D.Kouznetsov. Superexponential as special function. Vladikavkaz Mathematical Journal, 2010, v.12, issue 2, p.31-45.
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 06:14, 1 December 2018 |  | 3,341 × 1,675 (1.89 MB) | Maintenance script (talk | contribs) | Importing image file |
You cannot overwrite this file.
File usage
There are no pages that use this file.